[Scala MOOC I] Lec1: Functions & Evaluation

In this week, we'll learn the difference between functional imperative programming. We step through the basics of Scala; covering expressions, evaluation, conditionals, functions, and recursion

1.1 - Programming Paradigms

imperative programming:

• modify mutable variables
• using assignments
• control structures: if-else, loops, break, continue, return, etc.

~~~> Von Neumann computer:

conceptualize data structures word-by-word. ⇒ need higher level abstractions (theories).

theory doesn't describe mutations!
ex. theories of polynomials

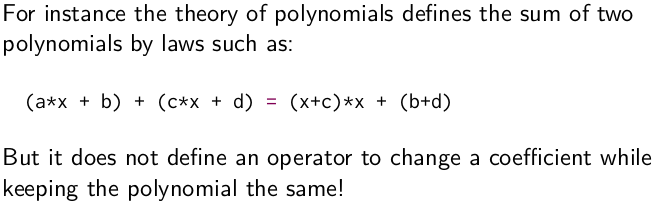
To implement high-level concepts following their math theories, there's no place for mutation.

Functional programming

functions in FP language are 1st-class citizens.

1.2 - Elements of Programming

in a language:

• primitive expressions
• operators to combin expressions
• abstract expressions: introduce a name for an expression

primitive types in scala are written capatilized:

REPL

interactive shell: write expressions and respond its value

Evaluation

evaluation: expression → value
evaluation stops onces it results in a value.

evaluate non-primitive expressions:

• take the left-most operator
• take (left and right) operands
• apply the operator to operands

evaluate names:
replace the name with the rhs of its definition.

definitions can have parameters.
ex. variable/return types are after their names, seperated by a :
def power(x: Double, y: Int): Double = ...

evaluate a parameterized function:

This scheme is called "substition model" — formalized in the lambda calculus. This can be applied as long as there is no side effects(ex. modify variable c++).

Termination
Q: does every expression evaluates to a value?
NO, ex.
def loop: Int = loop

change evaluation strategy
do not reduce argument values before rewrite function application.

call-by-name and call-by-value

the above evaluation schemes.

• call-by-value: reduce argument values before rewrite function application.

advantage: it evaluates every function argument only once.

• call-by-name: do not reduce arg values

advantage: a function argument is not evaluated if the corresponding parameter is unused in the evaluation
of the function body.

ex.

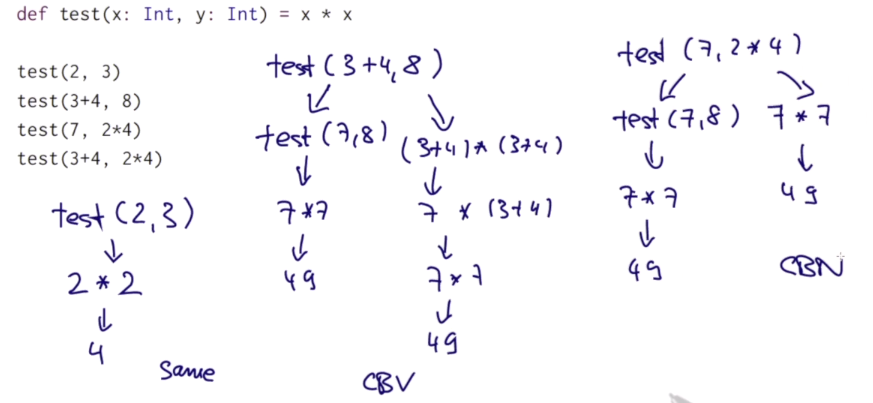
1.3 - Evaluation Strategies and Termination

CBV and CBN:

ex.

def first(x: Int, y: Int) = x  
first(1, loop)

in scala:

• normally use call-by-value (more efficient than call-by-name because it avoid repeated computation of CBN).
• but: if a => before a parameter type, that parameter is called by-name.

ex.

def constOne(x: Int, y: => Int) = 1  
constOne(1+2, loop)  
constOne(loop, 1)

1.4 - Conditionals and Value Definitions

conditional expressions

the if-else expression
the if-else is an expression, not statement.
ex. def abs(x: Int): Int = if (x>=0) x else -x
the x>=0 is a boolean expression, sometimes called predicates.

CBN and CBV for definition

• def form is by name, its rhs is evaluated at each use
• val is by value, evaluted at the definiton

ex.

scala> def loop:Boolean = loop  
loop: Boolean

scala> def x = loop  
x: Boolean

scala> val x = loop  
Execution interrupted by signal.

exercice: implement and(x,y)

scala> def and(x:Boolean, y: => Boolean) = if(x) y else false  
and: (x: Boolean, y: => Boolean)Boolean

scala> and(false, loop)  
res3: Boolean = false

1.5 - Example: square roots with Newton's method

def sqrt(x: Double): Double = ...

need helper (recursive) functions.

note: in scala the return type of recursive functions must be given.

def improve(guess: Double, x: Double): Double =  
  (x / guess + guess) / 2.0

def isGoodGuess(guess: Double, x: Double): Boolean =  
  math.abs((guess * guess - x)/x)<0.001

def sqrtItr(guess: Double, x: Double): Double = {  
  if (isGoodGuess(guess, x)) guess  
  else sqrtItr(improve(guess, x), x)  
}

def sqrt(x:Double): Double = sqrtItr(1, x)

1.6 - Blocks and Lexical Scope

nested functions

put aux functions inside a function.

def sqrt(x:Double): Double = {  
  def improve(guess: Double, x: Double): Double =  
    (x / guess + guess) / 2.0

  def isGoodGuess(guess: Double, x: Double): Boolean =  
    math.abs((guess * guess - x)/x)<0.001

  def sqrtItr(guess: Double, x: Double): Double = {  
    if (isGoodGuess(guess, x)) guess  
    else sqrtItr(improve(guess, x), x)  
  }

  sqrtItr(1, x)  
}

blocks and visibility

blocks: delimited by braces {...}
The last element of a block is an expression that defines its value.
Blocks are themselves expressions; a block may appear everywhere an expression can.

ex.

val x = 0  
def f(y: Int) = y +1  
val result = {  
  val x = f(3);  
  x * x  
} + x // result = 16

ex. eliminate the parameter x of helper functions inside the sqrt function

def sqrt(x:Double): Double = {  
  def improve(guess: Double): Double =  
    (x / guess + guess) / 2.0

  def isGoodGuess(guess: Double): Boolean =  
    math.abs((guess * guess - x)/x)<0.001

  def sqrtItr(guess: Double): Double = {  
    if (isGoodGuess(guess)) guess  
    else sqrtItr(improve(guess))  
  }

  sqrtItr(1)  
}

about multiline expressions: put the operator in the first line to avoid ambguity.

1.7 - Tail Recursion

review: evaluation of function application.

ex1. gcd

def gcd(a:Int, b:Int): Int=  
  if(b==0) a else gcd(b, a%b)

ex2. factorial

def factorial(n:Int): Int =  
  if(n==0) 1 else n*factorial(n-1)

difference from the gcd case:

• in gcd, the expression is reduced to a gcd()
• in factorial, add one more element to the expression in each step!

tail recursion

if a function calls itself as its last action, then the function's stack can be reused.
⇒ tail recursion functions are iterative process.

In general, if the last action of a function consists of calling a function (which may be the same), one stack frame would be sufficient for both functions. Such calls are called tail-calls.

tail-recursive function:

• more efficient
• can avoid stackoverflow
• might not be as clear as a non-tailrec version

add @tailrecannotation to the function, if its not tailrec, an error will be shown.

@tailrec  
def gcd(a: Int, b: Int): Int = ...

exercice: turn factorial into tailrec fashion.
→ idea: use an accumulator (partial result) acc .

def factorialTR(n:Int):Int = {  
  @tailrec  
  def fact(n:Int, acc:Int):Int = {  
    if(n==0) acc  
    else fact(n-1, n*acc)  
  }  
  fact(n, 1)  
}

Programming Assignment: Recursion

三道递归的练习题...

  /**  
    * Exercise 1  
    */  
  def pascal(c: Int, r: Int): Int = {  
    if (c == 0 || c == r) 1  
    else pascal(c, r - 1) + pascal(c - 1, r - 1)  
  }

  /**  
    * Exercise 2  
    */  
  def balance(chars: List[Char]): Boolean = {  
    def balance_rec(chars: List[Char], acc: Int): Boolean = {  
      if (acc<0) false  
      else if (chars.isEmpty) acc == 0  
      else if (chars.head == '(') balance_rec(chars.tail, acc+1)  
      else if (chars.head == ')') balance_rec(chars.tail, acc-1)  
      else balance_rec(chars.tail, acc)  
    }

    balance_rec(chars, 0)  
  }

  /**  
    * Exercise 3  
    */  
  def countChange(money: Int, coins: List[Int]): Int = {  
    if(money==0 ) 1  
    else if(coins.isEmpty || money<0) 0  
    else countChange(money, coins.tail) + countChange(money-coins.head, coins)  
  }